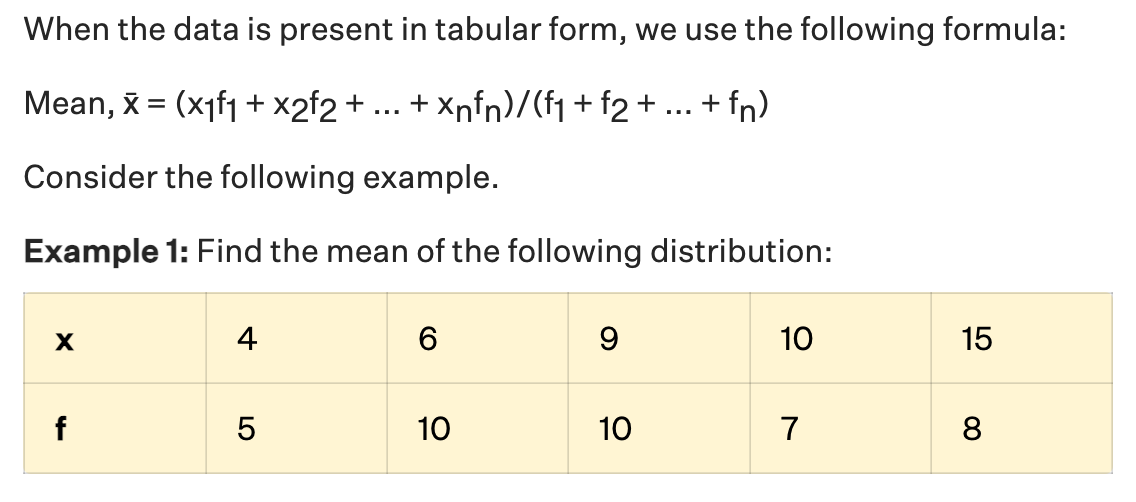
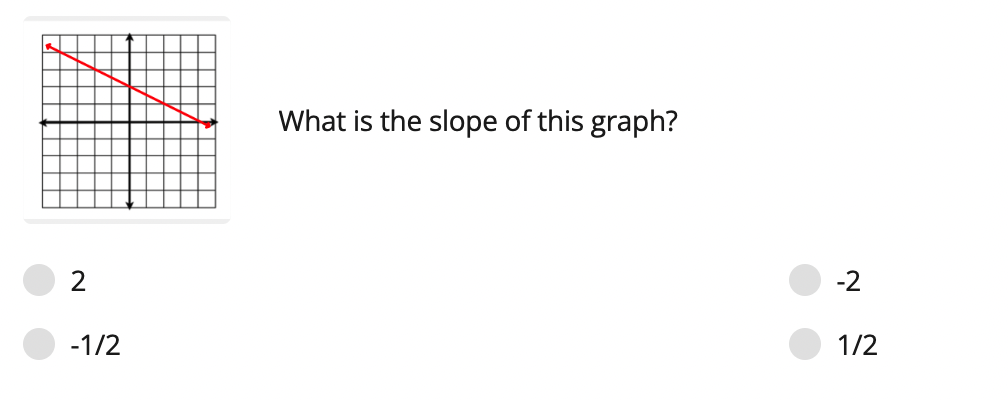The Ultimate Guide to Year 10 Mathematics

Wondering what Year 10 Maths has coming up for your child? Look no further! 

Year 10 sets the foundation for the Mathematics course that your child will choose for Year 11 and Year 12, so it is one of the most important years. It is especially crucial that they understand the concepts and skills that are being taught this year so that they can build on them for more complex mathematical skills. 

Number and Algebra

• Money and Financial Mathematics
  When working with Money and Financial Mathematics, students will learn how to connect compound interest formulas to repeated applications of simple interest using appropriate digital technologies. They will work with real information, data and interest rates to calculate compound interest and solve related problems. 

• Patterns and Algebra
  Your child will learn how to factorise algebraic expressions, simplify algebraic products and quotients using index laws and apply the four operations to simple algebraic fractions with numerical denominators. Students will also learn how to expand binomial products and factorise monic quadratic expressions using a variety of strategies.

  • Listed below are the formulas of algebraic expressions.

    • (a+b)2=a2+2ab+b2(𝑎+𝑏)2=𝑎2+2𝑎𝑏+𝑏2

    • (a−b)2=a2−2ab+b2(𝑎−𝑏)2=𝑎2−2𝑎𝑏+𝑏2

    • a2−b2=(a+b)(a−b)𝑎2−𝑏2=(𝑎+𝑏)(𝑎−𝑏)

    • a3+b3=(a+b)(a2−ab+b2)𝑎3+𝑏3=(𝑎+𝑏)(𝑎2−𝑎𝑏+𝑏2)

    • a3−b3=(a−b)(a2+ab+b2)𝑎3−𝑏3=(𝑎−𝑏)(𝑎2+𝑎𝑏+𝑏2)

    • (a+b)3=a3+3a2b+3ab2+b3(𝑎+𝑏)3=𝑎3+3𝑎2𝑏+3𝑎𝑏2+𝑏3

    • (a−b)3=a3−3a2b+3ab2−b3

• Linear and Nonlinear Relationships
  Lastly, through linear and non-linear relationships they will learn how to solve problems that include linear equations and how to graph various relations, including linear inequalities and simultaneous equations.

  Students will learn how to solve problems involving parallel lines and perpendicular lines, as well as explore the connection between algebraic and graphical relations of simple quadratics, circles and exponentials.

Measurement and Geometry 

Measurement and Geometry is split into three subtopics of using units of measurement, geometric reasoning, and Pythagoras theorem and trigonometry. Building on previous knowledge, students will continue to solidify their skills in solving problems involving surface area and volume for a range of prisms, cylinders and composite solids. 

Year 10s will also continue to learn how to set out and present their geometric reasoning. Your child will learn how to formulate proofs involving congruent triangles and angle properties. Additionally, they will learn how to apply logical reasoning by using congruence and similarity to apply to proofs involving plane shapes. 

Lastly, your child will extend their understanding of Pythagoras and trigonometry by solving right-angled triangle problems, including those involving direction, angles of elevation and depression. 

Statistics and Probability 

Chance

In the subtopic of Chance, your child will learn how to describe the results of two and three-step chance experiments, both with and without replacements, assign probabilities to outcomes and determine probabilities of events.
Additionally, they will learn how to use language like ‘if’, ‘then’, ‘given’, ‘of’, and ‘knowing that’ to investigate conditional statements and identify common mistakes in interpreting such language. 

Data Representation and Interpretation

Lastly, in Data Representation and Interpretation students will learn about various graphs, some of which they have encountered in earlier years. They will learn about how to determine the interquartile range, construct and interpret box plots to compare data sets and use scatter plots to investigate and comment on relationships between two numerical variables.

Your child will learn how to investigate and describe bivariate data where the independent variable is time and learn how to evaluate statistical reports in media by linking claims to displays, statistics and representative data. 

What should I do to improve my marks in Maths?

Students should set up a consistent study rhythm where they have time set aside each week for:

• Learning theory

• Practising methods

• Testing themselves

• Logging their mistakes

It is important that you keep track of your mistakes. Keeping a journal of mistakes will allow students to reflect on their errors and develop strategies to avoid making mistakes in the future.

Are you having trouble staying focused? Here are some ways to improve your productivity!